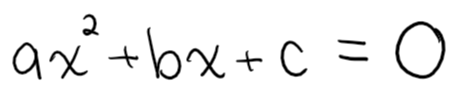Quadratic Equations: Like driving a car
After successfully completing the typical high-school unit on quadratic equations, students may jump into the process of solving a quadratic equation automatically and without having to think about it. However, the steps are made up of so many smaller components, each one requiring its own understanding.
To solve a quadratic equation (in particular, a quadratic equation written in standard form), you must complete the following steps – each of which requires its own set of knowledge and skills:
- Recognize that the equation is a quadratic, and that it is written in standard form.
- Factor the equation. This involves:
- Determining which method(s) to use to factor the equation. This requires knowledge of what the different possible factoring methods are, and when they should be applied.
- Correctly executing the factoring of the equation. This requires a thorough understanding of factors/multiples, as well as the basic arithmetic operations multiplication, addition, subtraction, and potentially division.
- Knowing when to stop, i.e. when the equation has been fully factored.
- Use the zero-product property to solve the equation. This involves:
- Understanding what the zero-product property is and how you can apply it to a factored equation
- Being able to solve linear equations for an unknown variable (this again takes knowledge of balancing equations, and basic arithmetic operations).
- Write the solutions of the equation. For this, you need to know:
- What a solution to an equation in one variable is
- How you obtain the solution from solving a linear equation
- Recognizing when there are no real solutions, and when two solutions are the same one
Example for "Quadratic Equations: Like driving a car":
https://bank.ecampusontario.ca/wp-content/uploads/2021/06/Quadratic-Equation.png